Inherent Errors in Current Core-Flooding Relative Permeability Data for Modelling Underground Hydrogen Storage

Abstract

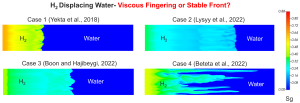
In the design and optimization of an underground hydrogen (H₂) storage facility in an aquifer or other reservoir system, the H₂/water relative permeabilities (RP) are the most critical two-phase data for input to numerical simulation. In this paper, we present a critical analysis of the published experimental H₂/water RP functions in the literature. We present fine-grid simulations of H₂ displacing water (denoted H₂ --> water) using three of the most widely cited steady-state RP datasets (13, 20, 38) in a mildly heterogeneous permeability field, at a field length scale of ~100m. Since the viscosity ratio between water and H₂ is (µ_w/µ_H2) ≈ 70, then at some length scale above a few meters, it is inevitable that the system must show immiscible viscous fingering of the H₂ into the water phase. Indeed, the emergence of viscous fingering at some length scale is a “sense check” that the input data used in the simulations are correct, especially the H₂/water relative permeability functions. 

In fact, none of the three published H₂/water RP curves leads to viscous fingering. Instead, they all show stabilized flood fronts. The reasons for this are due to shortcomings of the (conventional) gas/liquid experimental methods used to obtain the RP functions. These methods yield RP functions at the wrong force balance between the capillary, gravity and viscous forces. For fingering to emerge, it is necessary to derive the viscous dominated RP functions. An alternative, more physically appropriate set of viscous dominated “fingering RP functions” is proposed and applied. When applied at the core scale, these new RP functions show fully dispersed flow, as they do when applied in a vertical (downwards) gravity stable displacement. However, the viscous fingering emerges naturally in horizontal flow as the length scale of the system increases and viscous forces become dominant.