Modeling Desiccation Cracks in Opalinus Clay at Field Scale with the Phase-Field Approach
DOI:
https://doi.org/10.69631/ipj.v1i1nr8Keywords:
Desiccation, Opalinus Clay, Porous Media, Hydro-mechanical modeling, Fracture modelingAbstract
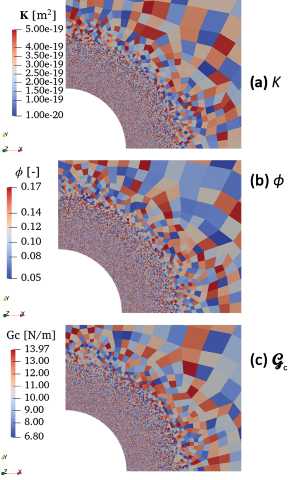
Geological materials such as Opalinus Clay show complex coupled hydro-mechanical behavior at laboratory and field scales. In the context of radioactive waste disposal, in-situ excavations might remain open for ventilation and operation for decades and, consequently, be susceptible to environmental changes such as desaturation. The saturation changes can then lead to mechanical deformation and desiccation cracks. To account for desiccation cracking at field scale, this study proposes an unsaturated hydro-mechanical model combined with the phase-field approach. Using laboratory and in-situ experimental data as input in the numerical model, the modeling framework is applied for simulating the hydro-mechanical effects and desiccation cracks reported in the Cyclic Deformation (CD-A) experiment carried out in the Opalinus Clay formation at the Mont Terri Rock Laboratory in Switzerland. Simulations with homogeneous and heterogeneous material properties generated from experimentally obtained ranges are carried out. Crack initiation and propagation show a good correlation with the monitored relative humidity range of the experiment. Practical information is summarized to motivate the application of the proposed formulation at different setups. Finally, possibilities to improve the framework and to reason simplification of more abstract models are indicated.
Downloads
References
Ambati, M., Gerasimov, T., & De Lorenzis, L. (2015). Phase-field modeling of ductile fracture. Computational Mechanics, 55(5), 1017–1040. https://doi.org/10.1007/s00466-015-1151-4 DOI: https://doi.org/10.1007/s00466-015-1151-4
Amor, H., Marigo, J.-J., & Maurini, C. (2009). Regularized formulation of the variational brittle fracture with unilateral contact: Numerical experiments. Journal of the Mechanics and Physics of Solids, 57(8):1209 – 1229. https://doi.org/10.1016/j.jmps.2009.04.011 DOI: https://doi.org/10.1016/j.jmps.2009.04.011
Balay, S., Abhyankar, S., Adams, M. F., et al. (2019a). PETSc Users Manual. Technical Report ANL-95/11 - Revision 3.11, Argonne National Laboratory. https://publications.anl.gov/anlpubs/2019/12/155920.pdf
Bilke, L., Fischer, T., Naumov, D., et al. (2022 April). OpenGeoSys, Zenodo, version 6.4.3. https://doi.org/10.5281/zenodo.7092676
Bilke, L., Flemisch, B., Kalbacher, T., et al. (2019). Development of open-source porous media simulators: Principles and experiences. Transport in Porous Media, 130(1), 337–361. https://doi.org/10.1007/s11242-019-01310-1 DOI: https://doi.org/10.1007/s11242-019-01310-1
Bock, H. (2009, June 30). RA Experiment. Updated review of the rock mechanics properties of the Opalinus Clay of the Mont Terri URL based on laboratory and field testing. Unpublished Manuscript, Mont Terri Project, TR 2008-04. https://www.mont-terri.ch/en/documentation/technical-reports.detail.document.html/mont-terri-internet/en/documents/technical-reports/TR2008-04.pdf.html
Bossart, P., Bernier F., Birkholzer J., et al. (2018). Mont Terri rock laboratory, 20 years of research: introduction, site characteristics and overview of experiments. In: Bossart, P., Milnes, A. (eds) Mont Terri Rock Laboratory, 20 Years. Swiss Journal of Geosciences Supplement, vol 5. Birkhäuser, Cham. https://doi.org/10.1007/978-3-319-70458-6_1 DOI: https://doi.org/10.1007/978-3-319-70458-6
Bourdin, B. (2007). Numerical implementation of the variational formulation for quasi-static brittle fracture. Interfaces and Free Boundaries, 411–430. https://doi.org/10.4171/IFB/171 DOI: https://doi.org/10.4171/ifb/171
Bourdin, B., Francfort, G. A., & Marigo, J.-J. (2000). Numerical experiments in revisited brittle fracture. Journal of the Mechanics and Physics of Solids, 48(4), 797–826. https://doi.org/10.1016/S0022-5096(99)00028-9 DOI: https://doi.org/10.1016/S0022-5096(99)00028-9
Bourdin, B., Francfort, G. A., & Marigo, J.-J. (2008). The variational approach to fracture. Journal of Elasticity, 91(1–3), 5–148. https://doi.org/10.1007/s10659-007-9107-3 DOI: https://doi.org/10.1007/s10659-007-9107-3
Bourdin, B., Marigo, J.-J., Maurini, C., et al. (2014). Morphogenesis and propagation of complex cracks induced by thermal shocks. Physical Review Letters, 112(1):1–5. https://doi.org/10.1103/PhysRevLett.112.014301 DOI: https://doi.org/10.1103/PhysRevLett.112.014301
Brooks, R. H., & Corey, A. T. (1964). Hydraulic properties of porous media. Hydrology papers, Vol. 3, Colorado State University, Fort Collins, USA. https://www.engr.colostate.edu/ce/facultystaff/yevjevich/papers/Yevjevich_n3_1964.pdf
Burke, S., Ortner, C., & Süli, E. (2013). An Adaptive Finite Element Approximation of a Generalized Ambrosio–Tortorelli Functional. Mathematical Models and Methods in Applied Sciences, 23(09):1663– 1697. https://doi.org/10.1142/S021820251350019X DOI: https://doi.org/10.1142/S021820251350019X
Cajuhi, T. (2019). Fracture in porous media: phase-field modeling, simulation and experimental validation. PhD thesis[Doctoral Disserstation, Technische Universität Braunschweig]. https://leopard.tu-braunschweig.de/servlets/MCRFileNodeServlet/dbbs_derivate_00045168/Diss_Cajuhi_Tuanny.pdf
Cajuhi, T., Haghighat, N., Maßmann, J., et al (2023). Hydro-Mechanical Effects and Cracking in Opalinus Clay. In: Kolditz, O., et al. GeomInt—Discontinuities in Geosystems from Lab to Field Scale. SpringerBriefs in Earth System Sciences. Springer, Cham. https://doi.org/10.1007/978-3-031-26493-1_2 DOI: https://doi.org/10.1007/978-3-031-26493-1_2
Cajuhi, T., Maßmann, J., & Ziefle, G. (2021a). Building the bridge between safety requirements and numerical modeling: an example considering crack development of Opalinus clay in laboratory and field scales. Safety of Nuclear Waste Disposal, 1: 165–167. https://doi.org/10.5194/sand-1-165-2021 DOI: https://doi.org/10.5194/sand-1-165-2021
Cajuhi, T., Sanavia, L., & De Lorenzis, L. (2018). Phase-field modeling of fracture in variably saturated porous media. Computational Mechanics, 61(3): 299-318. https://doi.org/10.1007/s00466-017-1459-3 DOI: https://doi.org/10.1007/s00466-017-1459-3
Cajuhi, T., Ziefle, G., Maßmann, J., et al. (2021b). Numerical modeling of hydro-mechanical coupled effects in the cyclic deformation (CD-A) experiment: First results and comparison with observations. EGU21, the 23rd EGU General Assembly, held online 19-30 April, 2021, id.EGU21-4173. https://doi.org/10.5194/egusphere-egu21-4173 DOI: https://doi.org/10.5194/egusphere-egu21-4173
Ehlers, W. & Luo, C. (2018). A phase-field approach embedded in the Theory of Porous Media for the description of dynamic hydraulic fracturing, Part II: The crack-opening indicator. Computer Methods in Applied Mechanics and Engineering, 341: 429–442. https://doi.org/10.1016/j.cma.2018.07.006 DOI: https://doi.org/10.1016/j.cma.2018.07.006
Francfort, G. & Marigo, J.-J. (1998). Revisiting brittle fracture as an energy minimization problem. Journal of the Mechanics and Physics of Solids, 46(8): 1319–1342. https://doi.org/10.1016/S0022-5096(98)00034-9 DOI: https://doi.org/10.1016/S0022-5096(98)00034-9
Freddi, F. & Royer-Carfagni, G. (2010). Regularized variational theories of fracture: a unified approach. Journal of the Mechanics and Physics of Solids, 58(8): 1154–1174. https://doi.org/10.1016/j.jmps.2010.02.010 DOI: https://doi.org/10.1016/j.jmps.2010.02.010
Fredlund, D. G. & Xing, A. (1994). Equations for the soil-water characteristic curve. Canadian Geotechnical Journal, 31(4): 521–532. https://doi.org/10.1139/t94-061 DOI: https://doi.org/10.1139/t94-061
Gao, H. & Rice, J. R. (1987). Somewhat circular tensile cracks. International Journal of Fracture, 33(3): 155–174. https://doi.org/10.1007/BF00013168 DOI: https://doi.org/10.1007/BF00013168
Garitte, B., Nguyen, T., Barnichon, J., et al. (2017). Modelling the Mont Terri HE-D experiment for the Thermal– Hydraulic–Mechanical response of a bedded argillaceous formation to heating. Environmental Earth Sciences, 76(9): 345. https://doi.org/10.1007/s12665-017-6662-1 DOI: https://doi.org/10.1007/s12665-017-6662-1
Gawin, D. and Schrefler, B. (1996). Thermo-hydro-mechanical analysis of partially saturated porous materials. Engineering Computations, 13(7): 113–143. https://doi.org/10.1108/02644409610151584 DOI: https://doi.org/10.1108/02644409610151584
Grunwald, N., Lehmann, C., Maßmann, J., et al. (2022). Non- isothermal two-phase flow in deformable porous media: systematic open-source implementation and verification procedure. Geomechanics and Geophysics for Geo-Energy and Geo-Resources, 8(3):107. https://doi.org/10.1007/s40948-022-00394-2 DOI: https://doi.org/10.1007/s40948-022-00394-2
Heider, Y. (2021). A review on phase-field modeling of hydraulic fracturing. Engineering Fracture Mechanics, 253: 107881. https://doi.org/10.1016/j.engfracmech.2021.107881 DOI: https://doi.org/10.1016/j.engfracmech.2021.107881
Heider, Y. & Markert, B. (2017). A phase-field modeling approach of hydraulic fracture in saturated porous media. Mechanics Research Communications, 80: 38–46. https://doi.org/10.1016/j.mechrescom.2016.07.002 DOI: https://doi.org/10.1016/j.mechrescom.2016.07.002
Heider, Y. & Sun, W. (2020). A phase field framework for capillary-induced fracture in unsaturated porous media: Drying-induced vs. hydraulic cracking. Computer Methods in Applied Mechanics and Engineering, 359: 112647. https://doi.org/10.1016/j.cma.2019.112647 DOI: https://doi.org/10.1016/j.cma.2019.112647
Hu, H., Braun, P., Delage, P., et al. (2021). Evaluation of anisotropic poroelastic properties and permeability of the Opalinus Clay using a single transient experiment. Acta Geotechnica, 16(4): 2131–2142. https://doi.org/10.1007/s11440-021-01147-3 DOI: https://doi.org/10.1007/s11440-021-01147-3
Hu, Z., Cajuhi, T., Toropovs, N., et al. (2023). A neutron radiography study on the drying of cement mortars: effect of mixture composition and crack length. Cement and Concrete Research, 172: 107245. https://doi.org/10.1016/j.cemconres.2023.107245 DOI: https://doi.org/10.1016/j.cemconres.2023.107245
Hun, D.-A. (2020). Fracture modeling in clay materials under hydric shrinkageModélisation de fissure dans les matériaux argileux sous retrait hydrique: numerical models, comparisons with experiments and stochastic aspects [Phd thesis]. Materials. Université Paris-Est. NNT: 2020PESC2054. tel-03238425. https://theses.hal.science/tel-03238425v1/file/79165_HUN_2020_archivage.pdf
Kafle, L., Xu W.-J., Zeng, S.-Y., et al. (2021). A numerical investigation of slope stability influenced by the combined effects of reservoir water level fluctuations and precipitation: A case study of the Bianjiazhai landslide in China. Engineering Geology, 297:106508. https://doi.org/10.1016/j.enggeo.2021.106508 DOI: https://doi.org/10.1016/j.enggeo.2021.106508
Kneuker, T. & Furche, M. (2021). Capturing the structural and compositional variability of Opalinus Clay: constraints from multidisciplinary investigations of Mont Terri drill cores (Switzerland). Environmental Earth Sciences, 80, 421. https://doi.org/10.1007/s12665-021-09708-1 DOI: https://doi.org/10.1007/s12665-021-09708-1
Kolditz, O., Bauer, S., Bilke, L., et al. (2012). OpenGeoSys: an open-source initiative for numerical simulation of thermo-hydro-mechanical/chemical (THM/C) processes in porous media. Environmental Earth Sciences, 67(2):589–599. https://doi.org/10.1007/s12665-012-1546-x DOI: https://doi.org/10.1007/s12665-012-1546-x
Kolditz, O., Görke, U.-J., Konietzky, H., et al. (2021). GeomInt–Mechanical Integrity of Host Rocks. Series: Terrestrial Environmental Sciences. Springer, 2021. ISBN 978-3-030-61908-4, DOI: 10.1007/978-3-030-61909-1. DOI: https://doi.org/10.1007/978-3-030-61909-1
Lewis, R. W., Schrefler, B. A. (1999). The Finite Element Method in the Static and Dynamic Deformation and Consolidation of Porous Media (2nd ed). John Wiley & Sons. ISBN: 978-0-471-92809-6.
Lorenzis, L. D. & Maurini, C. (2021). Nucleation under multi-axial loading in variational phase-field models of brittle fracture. International Journal of Fracture, 237: 61-81. https://doi.org/10.1007/s10704-021-00555-6 DOI: https://doi.org/10.1007/s10704-021-00555-6
Luo, C., Sanavia, L., & Lorenzis, L. D. (2023). Phase-field modeling of drying-induced cracks: Choice of coupling and study of homogeneous and localized damage. Computer Methods in Applied Mechanics and Engineering, 410: 115962. https://doi.org/10.1016/j.cma.2023.115962 DOI: https://doi.org/10.1016/j.cma.2023.115962
Mandal, T. K., Nguyen, V. P., & Wu, J.-Y. (2019). Length scale and mesh bias sensitivity of phase-field models for brittle and cohesive fracture. Engineering Fracture Mechanics, 217: 106532. https://doi.org/10.1016/j.engfracmech.2019.106532 DOI: https://doi.org/10.1016/j.engfracmech.2019.106532
Marschall, P. & Giger, S. (2014). Nagra technical report 14-02, geological basics - Dossier IV - Information on geomechanics; SGT Etappe 2: Vorschlag weiter zu untersuchender geologischer Standortgebiete mit zugehörigen Standortarealen für die Oberflächenanlage -- Geologische Grundlagen -- Dossier IV -- Geomechanische Unterlagen. Technical report, National Cooperative for the Disposal of Radioactive Waste (NAGRA). ISSN 1015-2636; TRN: CH1701027088320.
Maßmann, J., Uehara, S.-I., Rejeb, A., et al. (2009). Investigation of desaturation in an old tunnel and new galleries at an argillaceous site. Environmental Geology, 57(6): 1337–1345. https://doi.org/10.1007/s00254-008-1438-2 DOI: https://doi.org/10.1007/s00254-008-1438-2
Maßmann, J., Ziefle, G., Costabel, S., et al. (2020). In-situ Experiment on the Influence of Humidity on the Cyclic and Long-Term Deformation Behavior (CD-A) of the Opalinus Clay at the Mont Terri Rock Laboratory, Switzerland: Excavation of the Twin Niches, First Measurements, Simulations and Analysis. In EGU General Assembly Conference Abstracts, page 9314. https://presentations.copernicus.org/EGU2020/EGU2020-9314_presentation.pdf DOI: https://doi.org/10.5194/egusphere-egu2020-9314
Meng, W., Wanqing, S., Jiangfeng, L., et al. (2022). Phase-field modeling of cracking process in partially saturated porous media and application to rainfall-induced landslides. Engineering Geology, 310: 106884. https://doi.org/10.1016/j.enggeo.2022.106884 DOI: https://doi.org/10.1016/j.enggeo.2022.106884
Mesgarnejad, A., Bourdin, B., & Khonsari, M. M. (2015). Validation simulations for the variational approach to fracture. Computer Methods in Applied Mechanics and Engineering, 290: 420–437. https://doi.org/10.1016/j.cma.2014.10.052 DOI: https://doi.org/10.1016/j.cma.2014.10.052
Miehe, C., Welschinger, F., & Hofacker, M. (2010). Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations. International Journal for Numerical Methods in Engineering, 83(10):1273–1311. https://doi.org/10.1002/nme.2861 DOI: https://doi.org/10.1002/nme.2861
Mumford, D. & Shah, J. (1989). Optimal approximations by piecewise smooth functions and associated variational problems. Communications on Pure and Applied Mathematics, 42(5): 577–685. https://doi.org/10.1002/cpa.3160420503 DOI: https://doi.org/10.1002/cpa.3160420503
Nguyen, G. D. & Houlsby, G. T. (2007). Non-local damage modelling of concrete: a procedure for the determination of model parameters. International Journal for Numerical and Analytical Methods in Geomechanics, 31(7): 867–891. https://doi.org/10.1002/nag.563 DOI: https://doi.org/10.1002/nag.563
Nikolic, M., Ibrahimbegovic, A., & Miscevic, P. (2016). Discrete element model for the analysis of fluid-saturated fractured poro-plastic medium based on sharp crack representation with embedded strong discontinuities. Computer Methods in Applied Mechanics and Engineering, 298: 407–427. https://doi.org/10.1016/j.cma.2015.10.009 DOI: https://doi.org/10.1016/j.cma.2015.10.009
[OpenGeoSys]. (2020, May 4). MtTerri Wisdome [Video]. YouTube. https://www.youtube.com/watch?v=I1c8E77FEz0
Pham, K., Amor, H., Marigo, J.-J., et al. (2011). Gradient Damage Models and Their Use to Approximate Brittle Fracture. International Journal of Damage Mechanics, 20(4, SI): 618–652. https://doi.org/10.1177/1056789510386852 DOI: https://doi.org/10.1177/1056789510386852
Pitz, M., Kaiser, S., Grunwald, N., et al. (2023). Non-isothermal consolidation: A systematic evaluation of two implementations based on multiphase and Richards equations. International Journal of Rock Mechanics and Mining Sciences, 170: 105534. https://doi.org/10.1016/j.ijrmms.2023.105534 DOI: https://doi.org/10.1016/j.ijrmms.2023.105534
Regard, V., Schefer, S., Steiner, P., et al (2022). CD-A experiment: seasonal crack mapping in twin niches. Technical report, Mont Terri Technical Note 2021-63. https://extranet.mont-terri.ch/MONT_TERRI_BASIS/2_REPORTING/1_Technical_notes/Phase_26_TN2021/TN2021_63/TN2021_63.pdf
Richards, L. (1931). Capillary conduction of liquids through porous mediums. Physics, 1(5):318–333. https://doi.org/10.1063/1.1745010 DOI: https://doi.org/10.1063/1.1745010
Sanavia, L. and Schrefler, B. (2002). A finite element model for water saturated and partially saturated geomaterials: Space and time discretisation for a multiphase porous material model. Revue Française de Génie Civil, 6(6): 1083–1098. https://doi.org/10.1080/12795119.2002.9692733 DOI: https://doi.org/10.1080/12795119.2002.9692733
Santillán, D., Juanes, R., & Cueto-Felgueroso, L. (2017). Phase field model of fluid-driven fracture in elastic media: Immersed-fracture formulation and validation with analytical solutions. Journal of Geophysical Research: Solid Earth, 122(4):2565–2589. https://doi.org/10.1002/2016JB013572 DOI: https://doi.org/10.1002/2016JB013572
Schrefler, B. A., Simoni, L., & Markert, B. (2017). Multifield Problems. Part 2. Solids and Structures. Encyclopedia of Computational Mechanics Second Edition. 1–68. https://doi.org/10.1002/9781119176817.ecm2040 DOI: https://doi.org/10.1002/9781119176817.ecm2040
Shashank, M. & Song, X. (2019). Coupled Analysis of Desiccation Cracking in Unsaturated Soils Through a Non-Local Mathematical Formulation. Geosciences, 9(10): 428. https://doi.org/10.3390/geosciences9100428 DOI: https://doi.org/10.3390/geosciences9100428
Sicsic, P., Marigo, J.-J., & Maurini, C. (2014). Initiation of a periodic array of cracks in the thermal shock problem: a gradient damage modeling. Journal of the Mechanics and Physics of Solids, 63: 256–284. https://doi.org/10.1016/j.jmps.2013.09.003 DOI: https://doi.org/10.1016/j.jmps.2013.09.003
Stirling, R., Davie, C., & Glendinning, S. (2015). Multiphase modelling of desiccation cracking in the near-surface of compacted soils. Proceedings of the 16th European Conference on Soil Mechanics and Geotechnical Engineering. ICE Publishing: 2311–2316. https://eprints.ncl.ac.uk/225375
Taherdangkoo, R., Nagel, T., Tang, Anh Minh A. M., et al. (2022). Coupled Hydro-Mechanical Modeling of Swelling Processes in Clay–Sulfate Rocks. Rock Mechanics and Rock Engineering, 55(12): 7489-7501. 10.1007/s00603-022-03039-8. Hal-03905361. https://doi.org/10.1007/s00603-022-03039-8 DOI: https://doi.org/10.1007/s00603-022-03039-8
Tang, C.-S., Wang, D.-Y., Shi, B., et al. (2016). Effect of wetting–drying cycles on profile mechanical behavior of soils with different initial conditions. Catena, 139: 105–116. https://doi.org/10.1016/j.catena.2015.12.015 DOI: https://doi.org/10.1016/j.catena.2015.12.015
Tang, C.-S., Zhu, C., Cheng, Q., et al. (2021). Desiccation cracking of soils: A review of investigation approaches, underlying mechanisms, and influencing factors. Earth-Science Reviews, 216: 103586. https://doi.org/10.1016/j.earscirev.2021.103586 DOI: https://doi.org/10.1016/j.earscirev.2021.103586
Tanné, E., Bourdin, B., & Yoshioka, K. (2022). On the loss of symmetry in toughness dominated hydraulic fractures. International Journal of Fracture, 237(1-2):189–202. https://doi.org/10.1007/s10704-022-00623-5 DOI: https://doi.org/10.1007/s10704-022-00623-5
Tanné, E., Li, T., Bourdin, B., Marigo, J.-J., and Maurini, C. (2018). Crack nucleation in variational phase-field models of brittle fracture. Journal of Mechanical and Physics of Solids, 110: 80–99. https://doi.org/10.1016/j.jmps.2017.09.006 DOI: https://doi.org/10.1016/j.jmps.2017.09.006
Thomson, W. (1872). 4. On the Equilibrium of Vapour at a Curved Surface of Liquid. Proceedings of the Royal Society of Edinburgh, Volume 7: 63–68. https://doi.org/10.1017/S0370164600041729 DOI: https://doi.org/10.1017/S0370164600041729
van Dijk, N. P., Espadas-Escalante, J. J., & Isaksson, P. (2020). Strain energy density decompositions in phase-field fracture theories for orthotropy and anisotropy. International Journal of Solids and Structures, 196: 140–153. https://doi.org/10.1016/j.ijsolstr.2020.04.022 DOI: https://doi.org/10.1016/j.ijsolstr.2020.04.022
Van Genuchten, M. (1980). A Closed-Form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Science Society of America Journal, 44(5): 892–898. https://doi.org/10.2136/sssaj1980.03615995004400050002x DOI: https://doi.org/10.2136/sssaj1980.03615995004400050002x
Wang, H., de La Vaissière, R., Vu, M.-N., et al. (2022a). Numerical modelling and in-situ experiment for self-sealing of the induced fracture network of drift into the Callovo-Oxfordian claystone during a hydration process. Computers and Geotechnics, 141:1 04487. https://doi.org/10.1016/j.compgeo.2021.104487 DOI: https://doi.org/10.1016/j.compgeo.2021.104487
Wang, H., Dong, Q., de La Vaissière, R. et al. Investigation of Hydro-mechanical Behaviour of Excavation Induced Damage Zone of Callovo-Oxfordian Claystone: Numerical Modeling and In-situ Experiment. Rock Mechanics and Rock Engineering, 55: 6079–6102. https://doi.org/10.1007/s00603-022-02938-0 DOI: https://doi.org/10.1007/s00603-022-02938-0
Wild, K. & Amann, F. (2018). Experimental study of the hydro-mechanical response of Opalinus Clay - Part I: Pore pressure response and effective geomechanical properties under consideration of confinement and anisotropy. Engineering Geology, 237: 32–41. https://doi.org/10.1016/j.enggeo.2018.02.012 DOI: https://doi.org/10.1016/j.enggeo.2018.02.012
Wild, K. M., Walter, P., & Amann, F. (2017). The response of Opalinus Clay when exposed to cyclic relative humidity variations. Solid Earth, 8: 351–360. https://doi.org/10.5194/se-8-351-2017 DOI: https://doi.org/10.5194/se-8-351-2017
Yan, H., Jivkov, A. P., & Sedighi, M. (2022). Modelling soil desiccation cracking by peridynamics. Géotechnique, 73(5): 388-400. https://doi.org/10.1680/jgeot.21.00032 DOI: https://doi.org/10.1680/jgeot.21.00032
Yoshioka, K. & Bourdin, B. (2016). A variational hydraulic fracturing model coupled to a reservoir simulator. International Journal of Rock Mechanics and Mining Sciences, 88: 137–150. https://doi.org/10.1016/j.ijrmms.2016.07.020 DOI: https://doi.org/10.1016/j.ijrmms.2016.07.020
Yoshioka, K., Mollaali, M., & Kolditz, O. (2021). Variational phase-field fracture modeling with interfaces. Computer Methods in Applied Mechanics and Engineering, 384: 113951. https://doi.org/10.1016/j.cma.2021.113951 DOI: https://doi.org/10.1016/j.cma.2021.113951
Yoshioka, K., Naumov, D., and Kolditz, O. (2020). On Crack Opening Computation in Variational Phase-Field Models for Fracture. Computer Methods in Applied Mechanics and Engineering, 369: 113210. https://doi.org/10.1016/j.cma.2020.113210 DOI: https://doi.org/10.1016/j.cma.2020.113210
Yu, Z., Shao, J., Duveau, G., et al. (2021). Numerical modeling of deformation and damage around underground excavation by phase-field method with hydromechanical coupling. Computers and Geotechnics, 138: 104369. https://doi.org/10.1016/j.compgeo.2021.104369 DOI: https://doi.org/10.1016/j.compgeo.2021.104369
Zhang, C.-L. (2017). Response of clay rock to moisture change. Advances in Laboratory Testing and Modelling of Soils and Shales: 155–164. https://link.springer.com/chapter/10.1007/978-3-319-52773-4_17 DOI: https://doi.org/10.1007/978-3-319-52773-4_17
Zhang, C.-L., Komischke, M., Kröhn, M., et al (2019). Experimental study of the mechanical behaviour of the sandy facies of Opalinus Clay at Mont-Terri. LT-A programme within the Mont-Terri-Project. Technical report, Gesellschaft für Anlagen und Reaktorsicherheit (GRS) GmbH. ISBN 978-3-947685. https://inis.iaea.org/search/search.aspx?orig_q=RN:51064336
Zhang, J., Niu, G., Li, X., and Sun, D. (2020). Hydro-mechanical behavior of expansive soils with different dry densities over a wide suction range. Acta Geotechnica, 15(1): 265–278. https://dx.doi.org/10.1007/s11440-019-00874-y DOI: https://doi.org/10.1007/s11440-019-00874-y
Ziaei-Rad, V., Mollaali, M., Nagel, T., et al. (2022). Orthogonal decomposition of anisotropic constitutive models for the phase field approach to fracture. Journal of the Mechanics and Physics of Solids, 171: 105143. https://doi.org/10.1016/j.jmps.2022.105143 DOI: https://doi.org/10.1016/j.jmps.2022.105143
Ziefle, G., Cajuhi, T., Condamin, S., et al (2021). From process to system understanding with multi-disciplinary investigation methods: set-up and first results of the CD-A experiment (Mont Terri Rock Laboratory). Safety of Nuclear Waste Disposal, 1, pages 79–81. https://doi.org/10.5194/sand-1-79-2021 DOI: https://doi.org/10.5194/sand-1-79-2021
Ziefle, G., Cajuhi, T., Costabel, S., et al. (2023a). CD-A twin niches in the Mont Terri Rock Laboratory: Characterization and interpretation of hydraulic parameters with regard to safety aspects. International Journal of Rock Mechanics and Mining Sciences, 174: 105624. https://doi.org/10.1016/j.ijrmms.2023.105624 DOI: https://doi.org/10.1016/j.ijrmms.2023.105624
Ziefle, G., Cajuhi, T., Costabel, S., Furche, M., and Maßmann, J. (2024). Water content evolution in the EDZ of Opalinus Clay: A methodic approach for a comparative interpretation of measurements and modelling. Rock Mechanics and Rock Engineering. https://doi.org/10.1007/s00603-023-03717-1 DOI: https://doi.org/10.1007/s00603-023-03717-1
Ziefle, G., Cajuhi, T., Graebling, N., et al. (2022). Multi-disciplinary investigation of the hydraulic-mechanically driven convergence behaviour: CD-A twin niches in the Mont Terri Rock Laboratory during the first year. Geomechanics for Energy and the Environment, 31: 100325. https://doi.org/10.1016/j.gete.2022.100325 DOI: https://doi.org/10.1016/j.gete.2022.100325
Ziefle, G., Matray, J.-M., Maßmann, J., and Möri, A. (2018). Coupled hydraulic-mechanical simulation of seasonally induced processes in the Mont Terri rock laboratory (Switzerland). Swiss Journal of Geosciences, 110: 195-212. https://doi.org/10.1007/s00015-016-0252-1 DOI: https://doi.org/10.1007/s00015-016-0252-1
Zienkiewicz, O. C., Chan, A. H. C., Pastor, M., et al. (1999). Computational Geomechanics - With Special Reference to Earthquake Engineering. John Wiley & Sons. ISBN: 0-471-98285-7
Downloads
Additional Files
Published
Versions
- 2024-05-03 (2)
- 2024-04-26 (1)
Issue
Section
License
Copyright (c) 2024 Tuanny Cajuhi, Gesa Ziefle, Jobst Maßmann, Thomas Nagel, Keita Yoshioka

This work is licensed under a Creative Commons Attribution 4.0 International License.
Unless otherwise stated above, this is an open access article published by InterPore under either the terms of the Creative Commons Attribution-NonCommercial-NoDerivs 4.0 International License (CC BY-NC-ND 4.0) (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Article metadata are available under the CCo license.











